| Lecture
#5 |
 Textbook Textbook
Chapter 12 now No! No! No! No! No! No! |
| CURMUDGEON
GENERAL'S WARNING. These "slides"
represent highlights from lecture and are neither
complete nor meant to replace lecture. It is
advised not to use
these as a reliable means to replace missed
lecture material. Do so at risk to healthy
academic performance in 09-105. |
|
| Lecture outline |
Wave Nature of Matter Schrodinger's
Wave Equation applied to the hydrogen atom
Electron "spin"
ms, spin quantum number
Energies and geometries
Shapes
Heisenberg Uncertainty Principle
|
| If we go to four dimensions (x,y,z,t), a
fourth quantum number arises. |
 |
| The fourth quantum number is what is
familiarly referred to as the "spin" of an
electron. Spin can be pictured (erroneously, but with
little damage and much to be gained for convenience) as
being possible in either a clockwise or counterclockwise
sense...just two choices...sometimes referred to as
"up" and "down" pointed spins. |
 |
| The 1s wave function (or probability
amplitude) as a function of distance from the nucleus. |
 |
| The square of the 1s wave function or the
probability density of the electron in a 1s state |
 |
| The 2s wave function (or probability
amplitude) as a function of distance from the nucleus. |
 |
| The square of the 2s wave function or the
probability density of the electron in a 2s state |
 |
| The 3s wave function (or probability
amplitude) as a function of distance from the nucleus. |
 |
| The square of the 3s wave function or the
probability density of the electron in a 3s state |
 |
| The square of the 2pz wave
function or the probability density of the electron in a
2pz state. |
 |
| A comparison of the electron density
distribution for the 2s and 2p waves. The 2s has a larger
average radius than the 2p, but also a greater
probability of being found close to the nucleus. |
 |
| Shape and size of all the hydrogen 2p
contours |
 |
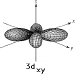
Shape and size of all the hydrogen 3d
contours.
|
 |
| Briefly...what is quantum mechanics? |
 |
| The Uncertainty Principle is an inherent
part of wave-particle duality |
 |
| The Uncertainty Principle (Section 12.5) |
 |
| An example calculation showing how
location in space and knowledge of momentum are
intertwined through wave-particle duality and the
Uncertainty Principle |
 |
| Conclusion of the calculation showing a
16% uncertainty in momentum is unavoidable |
 |
| A calculation exploring whether or not we
can improve our knowledge by using a higher resolution
probe of geometry. The uncertainty in momentum is
seriously worse than before. (The spread in velocities is
about 3 million m/s compared to the average of 1 million
m/s) |
 |
| With wave-particle duality, the
description of where a particle is moving -- its pathway
through space -- is meaningless. (Absolutely meaningless
in the realm where wavelengths are comparable to the size
of the system, like atoms and molecules, but unlike
baseball) We know only the region of space in which the
particle is probably found. We are obligated to
talk about probabilities. The concept of orbits, like
Bohr's planetary paths, are replaced with wave
"orbitals". The word orbitals is used
to mean the wave that describes a bound particle. |
 |
| The probability density measures what
fraction of the "electron" is found in a
cubical volume element around a particular point (x,y,z)
with respect to the nucleus. |
 |
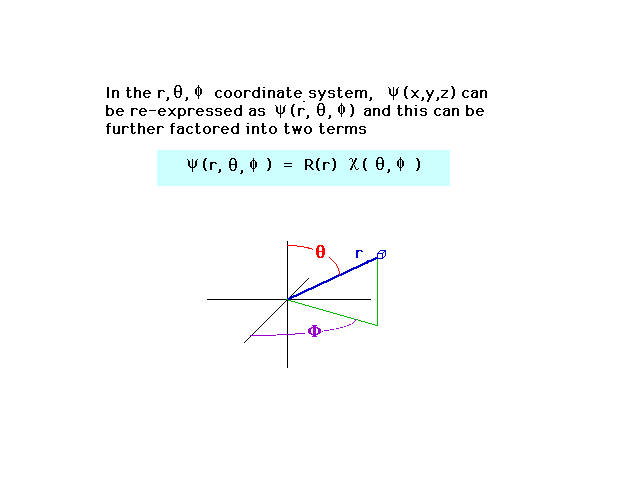
| We can also write the wave amplitude in
spherical coordinates. For a central nucleus, the wave
ammplitude then factors into two parts, one of which
depends only on the distance r between the nucleus and
electron. (You do not need to know the
blue-striped equation.) |
 |
| Another way of describing the density is
then by the fraction of the electrons distribution that
is in a spherical shell volume element at distance r from
the nucleus and covering all angles. (From the previous
slide, R is that part of the wave function that depends
only on distance from the nucleus, r.) |
 |
| A graphical comparison of the density
distribution (amplitude squared) and the radial
density distribution (amplitude squared multiplied by
r-squared) for a 1s orbital. The radial density
distribution graph is also referred to in the text as an
electron density plot. (p. 265) |
 |
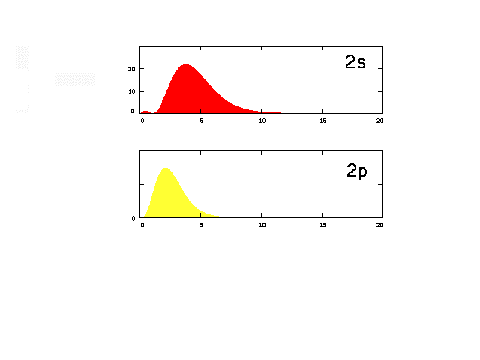
| The radial density distribution
for the n = 2 orbitals. |
 |
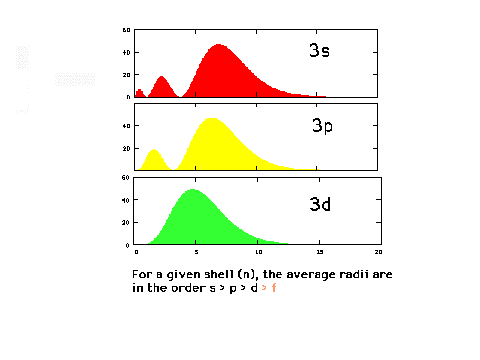
| The radial density distributions
for the n=3 orbitals. |
 |
| A close-up of the radial density
distribution near the nucleus. The blue "cloud"
represents where the inner, 1s electrons would most
likely be found. |
 |